How to calculate the square area of walls, floors or roofs for estimating

Calculating the square area of a square, rectangle, triangle & circle for pricing up quantities for DIY & carpentry jobs

You will often be required to calculate the square area of a floor, wall, roof or similar especially when estimating and pricing up Carpentry jobs.

If you need to use these formulas a lot for calculating various square areas and volumes, you could invest in a construction math calculator to speed things up.

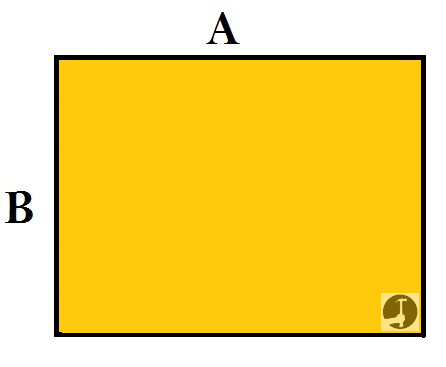
The formula and method to calculate the square area of a room like in the picture below is the same whether in centimetres, metres, feet, miles or any other measurement you need to use.

All you need to do is measure the length of the room (side A) and the width of the room (call it side B), making sure you keep to the same unit of measurement for each side.

Then, simply multiply the length (side A) by the width (side B).

For the two images below, assuming we want to find out the number of m2 (metres squared):

Length A (in metres) x Length B (in metres) = Metres squared

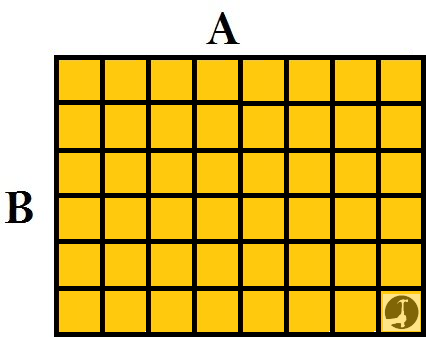
Therefore 8 x 6 = 48m2

You can count all of the squares in the grid on the right, there is definitely 48!

How to calculate the square area of a room

How to calculate the metre square of a room

In the example above although it is a rectangle shape, the sides were each a perfect whole number. The formula would work the same even if the measurements were decimals, for example 8.5 x 6.4 = 54.4 m2

When I am calculating the square area of a floor to work out how much laminate flooring to order I normally add between 5 - 10% extra to allow for cutting waste. To do this, multiply the answer by 1.05 or 1.1;

Answer x 1.05 = 5% extra added for waste

Answer x 1.10 = 10% extra added for waste

How to calculate the square area in feet and inches

Working out the square area in metric is fairly easy because it is in decimals.

There are a couple of different ways to calculate square area in feet and inches, which can require fractions instead and therefore gets more complicated.

Because one foot is made up of 12 inches (as opposed to 1 metre being made up of 100 centimetres) and each inch is split into fractions as well,

The easiest method I find is to convert the entire amount to just inches first;

For example if a rectangle had measurement of 13'4" by 17'8", I would multiply the first number for each side by 12 which would give the total number of inches in each full foot and then add the second number to it for the absolute total number of inches in length for each side.

To get a bit of a guide, we can quickly work out 13 x 17 = 221 sq feet, so we know we need to be a little more than that amount because of the extra inches involved.

Now for the correct amount of square feet and inches;

(13 x 12) + 4 = 160 inches (one side)

(17 x 12) + 8 = 204 inches (other side)

So the square area in inches, is 160 x 204 = 32,640 square inches

Now to work that back to the number of feet, divide by 144 (because a square foot is 144 inches squared);

32,640 / 144 = 226.66667 square feet (recurring)

This means the square area is 226 square feet, plus 2/3rds exactly of another foot.

Now to convert the decimal into fractions, 12 x 0.66667 = 8 inches.

So our square area is 226 feet & 8 inches or 226' 8".

(This is slightly higher than the 221 we arrived at by just multiplying the feet by each other first for a guide)

Click here to see more information about reading imperial measurements in feet and inches

How to calculate the square area of an L shaped room

When the room/wall/shape I want to work out the square area for isn't square but odd or L shaped like in the picture below, I separate the room into two or more manageable sections.

Imagine a line separating the two sections like the red one in the picture.

How to calculate the square area of a L shaped room

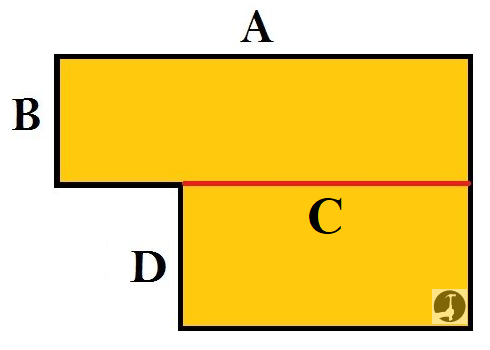
Then, treat these as two separate rooms and calculate the square area for both as in the first example.

After that, simply add the two answers together:

A x B = area in m2 (1)

C x D = area in m2 (2)

area (1) + area (2) = total area m2

How to calculate the square area of a right angle triangle

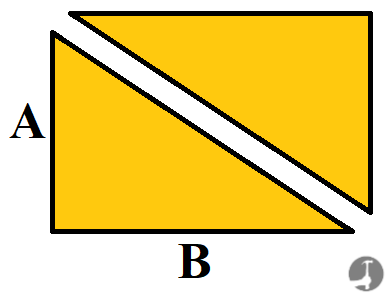
To calculate the square area of a right angle triangle, which you may need when calculating how much plywood you might need for a gable lean to roof for example, work it out the same as you would for a square or rectangle by first multiplying the length of the two sides at right angles to one another.

Then divide the answer by two, because two equal triangles make up the total rectangle.

For the triangle above;

(A x B) / 2 = square area of each triangle

For a full gable roof, the two triangles would make up a full rectangle shape and therefore you wouldn't need to halve the measurement, the off-cut/s could potentially be flipped and used to sheet the opposite gable.

How to calculate the volume & square area of a circle

Quite often as a carpenter I have needed the circle formulas information on this page to help calculate the amount of materials I need for floors for example and when forming arches for bricklayers.

If you are calculating the area of a round room in order to calculate how much flooring you will need, always allow a decent amount extra because there will unfortunately be more wastage than in a square room.

Calculate the square area & volume of a circle or tube

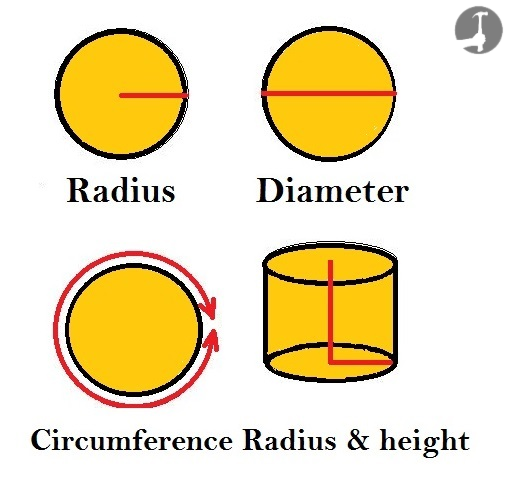
Radius = The distance from the outer edge to the centre.

Diameter = The furthest distance possible across the width (twice the length of the radius).

Circumference = Distance all the way round the outer edge. (I've only ever used that once to work out the length of skirting needed for a round room).

When making some of the calculations a number called Pi is often needed. Pi is a decimal value, that we will simplify to 3.14 for the purpose of these calculations.

These are some of the things you can calculate using Pi and the radius of a circle;